Sep 2025

Externally Dispersed Interferometry
Lawrence Livermore Nat. Lab.    &    Space Sciences Lab. UC Berkeley

EDI is a novel technique for the Doppler planet search
and high resolution spectroscopy

A method for boosting the Doppler sensitivity, spectral resolution, and lineshape stability of dispersive spectrographs, by adding a small interferometer into the beam and analyzing the fringe patterns

With the EDI, your inexpensive & compact spectrograph can now perform:
• Precision stellar Doppler radial velocimetry (paper1)(paper2) (planet detection! (Ge_Virgo_ApJ2006.pdf))
• High resolution spectroscopy: EDI boosts the spectral resolution of a dispersive spectrograph 2 - 20x over a wide simultaneous bandwidth by a process of heterodyning against the extremely periodic transmission comb of an interferometer, and combining the so-produced moire signals from one or more delays. (JATIS paper 2016 part 1, part 2).
 

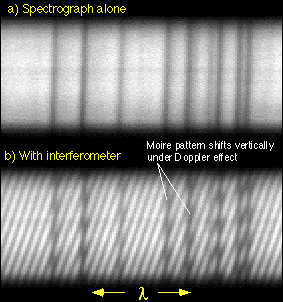
The EDI Method
An unequal-path Interferometer is added to a dispersive spectrograph

• The interferometer causes a periodic fringe vs wavelength

• The fringe pattern beats with the spectrum

• Heterodyning creates a moire pattern

• High-resolution features are measurable through the moire

• Nyquist limit and slit blurring are defeated

• The fringe fiducial allows error removal

• Phase stepping nulls instrumental errors

Solar spectrum without fringes (upper) and with fringes (lower).

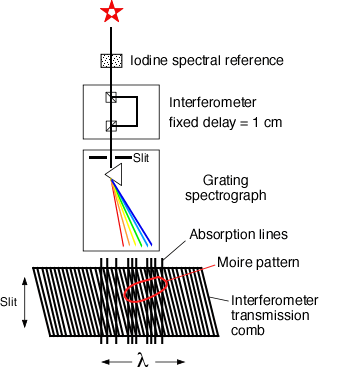
Doppler wavelength shifts are measured through phase shifts of "fringes" which form moire patterns seen in the spectrum. (The interferometer can't be used effectively alone because otherwise the fringes of many different wavelengths having many different phases would fall on the same detector pixels and wash itself out.) The grating spectrograph separates the fringes so that the fringe visibility can be high. But otherwise the key role of measuring a Doppler shift is with the interferometer.

The advantage of this is that the interferometer is independent of the thermomechanical instrument distortions that cause large velocity errors in the grating spectrograph.

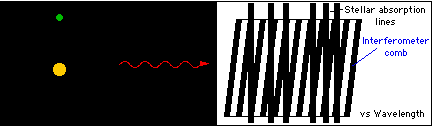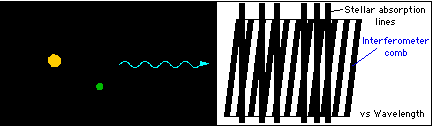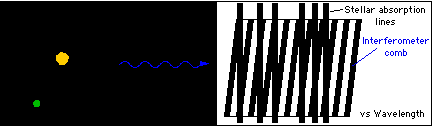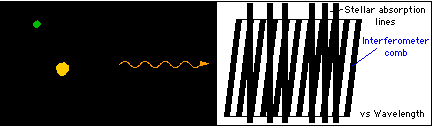
How EDI measures Doppler shifts

The interferometer is the "vernier", the grating the "coarse" readout
The EDI technique uses an interferometer for the fine wavelength discrimination and a grating spectrograph for the coarse discrimination. It is like the vernier and coarse readouts on a precision instrument. The interferometer has a much simpler instrument response, robust to many distortions that plague the grating.

The challenge: shifts are 500x smaller than feature linewidths!
When the exoplanet pulls the star it creates a Doppler shift in the spectrum vs wavelength. This shift is difficult to measure with a grating spectrograph because it is a small shift easily confused with irregularities typically found in the spectrograph.

Consider that the typical linewidth of a stellar absorption line is equivalent to 6000 m/s, so that a Jupiter-class exoplanet Doppler shift of 12 m/s is 500 times smaller than the width of the line! This is a very difficult measurement for a conventional spectrograph, and can be easily overwhelmed by uncontrolled distortions in the shape of its instrument response. While simultaneously measuring a spectral reference spectrum such as iodine reduces the effect of these irregularities, enough irregularities remain to make the measurement very challenging.

Our strategy is that the fundamental measurement, using the interferometer, has a mathematically simpler instrument response of a sinusoid, with only 3 degrees of freedom: phase, magnitude, and intensity offset. In contrast, the instrument response of a diffraction grating can have hundreds of degrees of freedom: one for each grating groove, which can vary with temperature and time and create significant velocity errors.

For both methods, these degrees of freedom must be calibrated away using a simultaneous spectral reference-- it's just easier to do this accurately when there are only three in the case of the interferometer.

More explanation on how EDI works for Doppler velocimetry is at this page.

How EDI measures High Resolution Spectra-- beyond disperser capability

Each measured moire pattern is mathematically processed to reverse the heterodyning effect, creating a wavelet.
The wavelet is the signal component in the input spectrum calculated to have formed the measured moire, for a given delay. (The data can be Fourier Transformed, shifted in frequency by the amount set by the delay, then inverse Fourier Transformed.) The input spectrum consists of a set of wavelets, in the Fourier sense that any function can be expressed as a sum of sinusoids. By summing the wavelets measured for a variety of delays, one can measure the input spectrum to many times higher resolution than with the disperser used alone. (This is related to the ability of a Fourier Transform Spectrometer, which is an isolated interferometer that scans its delay, to measure super high resolution with only a single pixel detector having zero spectral resolution).

Demonstration of high resolution spectroscopy with EDI at the Hale telescope, boosting the native resolution of the TripleSpec spectrograph about 8 times by combining wavelet signals from six delays. (a) ThAr lamp spectrum measured by conventional TripleSpec NIR spectrograph on the Cassegrain output of Hale Telescope. Its low resolution R=2000 (green cityscape indicating pixels) cannot resolve ThAr lamp doublet 7556, 7558 cm−1. (b) EDI reconstructed spectrum (thick red curve) equalized to R=16000 fully resolves doublet. Thin black curve is library spectrum. (c) EDI output spectrum (red) is sum of many wavelets, one per interferometer delay, with corrugation pitch proportional to delay value. Wavelets are measured moire ́ patterns mathematically shifted to higher frequency in Fourier space by the amount of each delay.

More explanation on how EDI boosts spectral resolution is at this page.

Site maintained by
David Erskine
derskine@spectralfringe.org